When you Assume you make an ASS out of U and ME (But sometimes it’s really useful for doing Maths and stuff)

In general assumptions are considered terrible. Don’t Assume, or you’ll make an ASS out of U and ME is something I’ve heard more than once in my life. But… Mathematical Assumptions are actually pretty useful. Consider the “Spherical cow existing in a vacuum”.

A Spherical cow in a vacuum is a shorthand way of saying that a physicists model of the real world has been simplified greatly in order to perform some analysis upon it. Simplifying things (like assuming a cow is spherical) allows physicists to do fun maths while ignoring complicated things like air drag, friction and cows using their farts as propulsion as they fly through the air.

But it’s not just in higher level physics that we start simplifying the world to make the maths easier.

We usually introduce students to mathematical assumptions very early on in their school life. The issue is that these assumptions are not usually explicitly talked about, rather they just become a part of doing the maths.

So what kinds of implicit assumptions do we ask students to make and rarely mention?

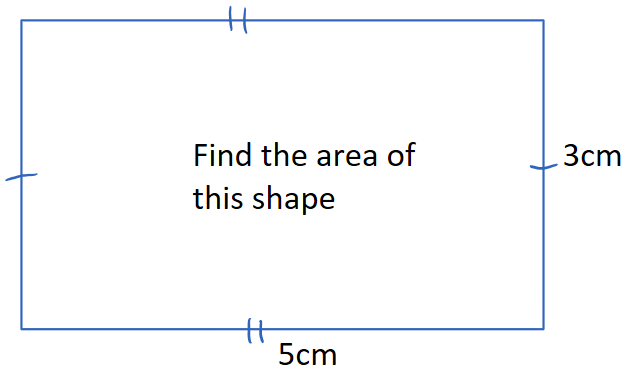
Consider the following question.

Nothing difficult here. The answer, of course, is…

Or is it?

Assumption 1. All numbers are exact

Who measured this particular rectangle? is it really 5cm, or is it 4.6cm and they just rounded up when they measured it? What if this image is identical to the image above, just with all measurements expressed to 2 decimal places.

Now this is an extreme to the point of absurd example. But it is equally absurd to assume that any length could be measured as exactly 5cm. The assumption of exact measurements is pretty wild, and we happily make it (and ask students to make it) every time we ask a maths question.

Assumption 2. Opposite Lengths are Equal

Perhaps you picked up from my wording of the question “Find the area of this shape”, that perhaps this shape isn’t what it appears to be. Well, it would be fairly inconvenient if the opposite sides weren’t equal, so I’m going to assume that they are!

Assumptions make maths possible, and in this question, assuming that the opposite sides are equal lengths is pretty useful, so let’s go with that. Also, assuming exact measurements is also pretty useful, so let’s go back to assuming that.

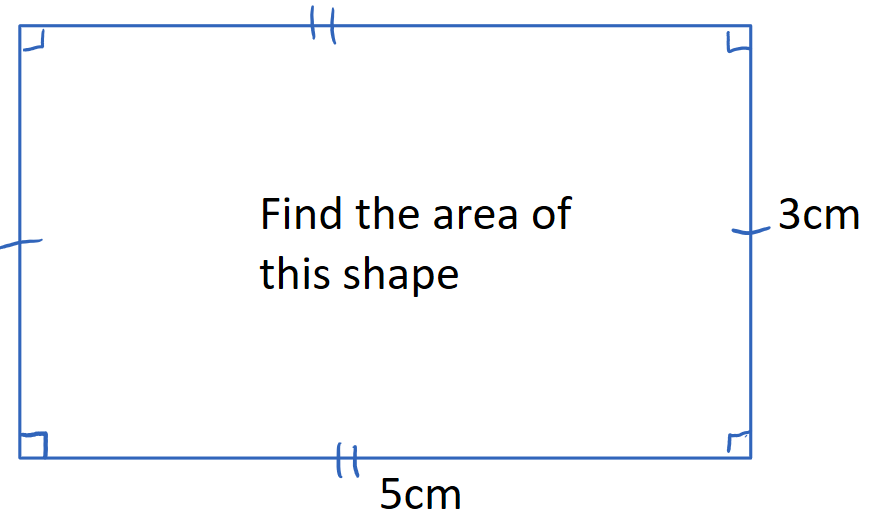
Assumption 3. All angles are 90 degrees

Let’s face it, it’s highly unlikely that this shape is NOT A RECTANGLE, and is in fact a parallelogram. How unlikely? Well, if I ASSUME (again) that the human eye perceives anywhere from 88 to 92 degrees as being a 90 degree angle, and I understand that there’s an infinite number of numbers between 88 and 92, the the probability of having exactly 90 degrees is 0 divided by infinity. 0%.

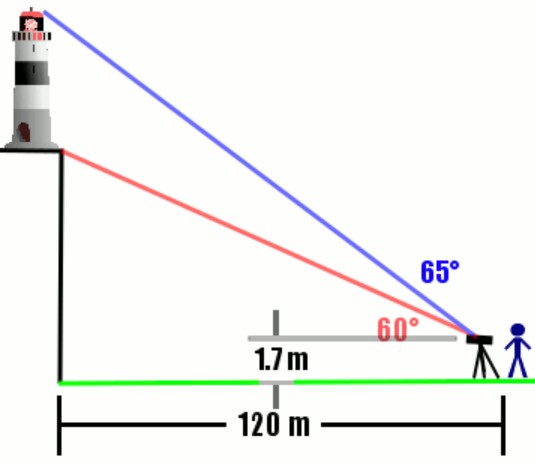
These 0% chance 90 degree angles show up everywhere in high school maths, particularly on the coast, where every cliff rises perpendicularly out of the calm blue ocean with a neat little lighthouse on top.

But, let’s continue assuming.

Assumption 4. Lines are infinitely thin

To a mathematician, the three questions above are all identical. To a man about to build a garden, these questions are very different.

Do you want the area around the outside edge of the blue line?
Do you want the area around the inside edge of the blue line?
Do you want the area of the blue line itself?

Infinitely thin lines is the greatest trick mathematicians ever pulled, and we do it ALL THE TIME!

Why am I talking about this?

The new QLD curriculum has a great little assessment called an Extended Modelling and Problem Solving Task. (It’s a mathematical report). One of the skills that students are marked on in this task is their ability to “documents appropriate mathematical assumptions”.

Teachers and students alike have struggled with what these assumptions are and why they are important. And the reason isn’t because it’s such a difficult concept. The reason is that it’s a concept that students and teachers have implicitly agreed upon from grade 1 all the way up to grade 12 and beyond.

Not only are assumptions important “because they’ll be on the test”, they’re also important because that’s how real world Maths is done. If you’re making your weekly budget, you’re going to have to make A LOT of assumptions about what you’ll spend and what you’ll make. If you’re an engineer and you’re figuring out how to design a bridge, you’re going to make a lot of assumptions about how it’s used and how it’s going to get built.

Examine all the Maths you do for the hidden assumptions you ask your students to make. Then explicitly talk about those assumptions with your students.

Because, if you ASSUME things without thinking about it, you’ll make an ASS out of U and ME. But if you ASSUME and you DO think about it… well that’s just good maths.